Review Unit 2a Slope and Slope Intercept Worksheet
Graphs
36 Use the Slope–Intercept Form of an Equation of a Line
Learning Objectives
By the end of this department, you will be able to:
- Recognize the relation between the graph and the gradient–intercept course of an equation of a line
- Place the slope and y-intercept grade of an equation of a line
- Graph a line using its slope and intercept
- Choose the most convenient method to graph a line
- Graph and interpret applications of slope–intercept
- Use slopes to identify parallel lines
- Use slopes to identify perpendicular lines
Recognize the Relation Between the Graph and the Slope–Intercept Form of an Equation of a Line
We have graphed linear equations by plotting points, using intercepts, recognizing horizontal and vertical lines, and using the bespeak–slope method. In one case we encounter how an equation in slope–intercept course and its graph are related, we'll have one more method we tin can employ to graph lines.
In Graph Linear Equations in 2 Variables, we graphed the line of the equation ![]() by plotting points. See (Figure). Allow'due south find the slope of this line.
by plotting points. See (Figure). Allow'due south find the slope of this line.
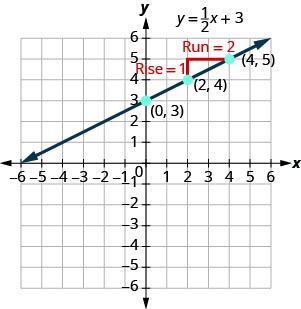
The red lines show u.s.a. the rise is 1 and the run is 2. Substituting into the slope formula:
![]()
What is the y-intercept of the line? The y-intercept is where the line crosses the y-axis, so y-intercept is ![]() . The equation of this line is:
. The equation of this line is:
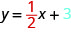
Notice, the line has:

When a linear equation is solved for ![]() , the coefficient of the
, the coefficient of the ![]() term is the slope and the constant term is the y-coordinate of the y-intercept. We say that the equation
term is the slope and the constant term is the y-coordinate of the y-intercept. We say that the equation ![]() is in slope–intercept form.
is in slope–intercept form.
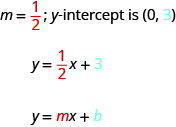
Slope-Intercept Form of an Equation of a Line
The gradient–intercept course of an equation of a line with slope ![]() and y-intercept,
and y-intercept, ![]() is,
is,
![]()
Sometimes the gradient–intercept form is called the "y-form."
Utilize the graph to discover the slope and y-intercept of the line, ![]() .
.
Compare these values to the equation![]() .
.
Use the graph to find the slope and y-intercept of the line ![]() . Compare these values to the equation
. Compare these values to the equation ![]() .
.
slope ![]() and y-intercept
and y-intercept ![]()
Use the graph to find the slope and y-intercept of the line ![]() . Compare these values to the equation
. Compare these values to the equation ![]() .
.
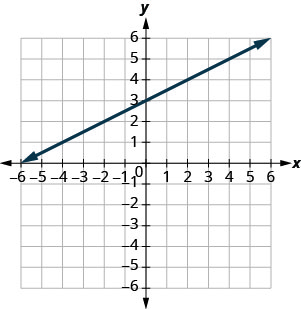
slope ![]() and y-intercept
and y-intercept ![]()
Place the Slope and y-Intercept From an Equation of a Line
In Empathize Slope of a Line, we graphed a line using the slope and a indicate. When we are given an equation in slope–intercept form, we can employ the y-intercept every bit the signal, and and so count out the slope from there. Let'south practise finding the values of the slope and y-intercept from the equation of a line.
Identify the slope and y-intercept of the line with equation ![]() .
.
Solution
Nosotros compare our equation to the slope–intercept form of the equation.
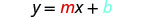 | |
| Write the equation of the line. | 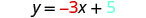 |
| Identify the slope. |  |
| Place the y-intercept. | 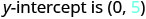 |
Place the gradient and y-intercept of the line ![]() .
.
![]()
Identify the gradient and y-intercept of the line ![]() .
.
![]()
When an equation of a line is not given in slope–intercept grade, our showtime footstep will exist to solve the equation for ![]() .
.
Identify the slope and y-intercept of the line with equation ![]() .
.
Identify the slope and y-intercept of the line ![]() .
.
![]()
Identify the slope and y-intercept of the line ![]() .
.
![]()
Graph a Line Using its Gradient and Intercept
Now that nosotros know how to observe the gradient and y-intercept of a line from its equation, we can graph the line past plotting the y-intercept and so using the gradient to find another indicate.
How to Graph a Line Using its Gradient and Intercept
Graph the line of the equation ![]() using its slope and y-intercept.
using its slope and y-intercept.
Graph the line of the equation ![]() using its gradient and y-intercept.
using its gradient and y-intercept.
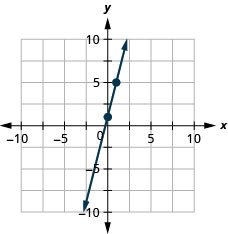
Graph the line of the equation ![]() using its slope and y-intercept.
using its slope and y-intercept.
Graph a line using its slope and y-intercept.
- Observe the slope-intercept form of the equation of the line.
- Identify the slope and y-intercept.
- Plot the y-intercept.
- Employ the slope formula
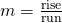 to identify the rise and the run.
to identify the rise and the run. - Starting at the y-intercept, count out the rise and run to marking the second point.
- Connect the points with a line.
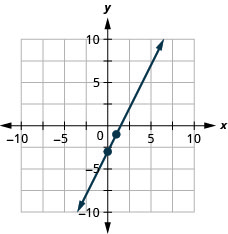
Graph the line of the equation ![]() using its slope and y-intercept.
using its slope and y-intercept.
Graph the line of the equation ![]() using its slope and y-intercept.
using its slope and y-intercept.
Graph the line of the equation ![]() using its slope and y-intercept.
using its slope and y-intercept.
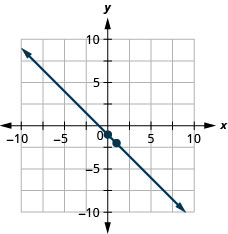
Graph the line of the equation ![]() using its gradient and y-intercept.
using its gradient and y-intercept.
Graph the line of the equation ![]() using its gradient and y-intercept.
using its gradient and y-intercept.
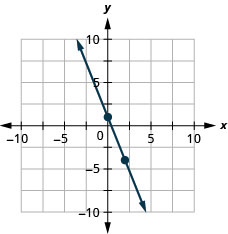
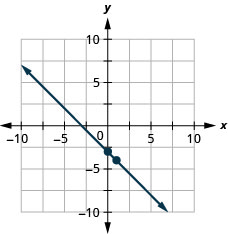
Graph the line of the equation ![]() using its slope and y-intercept.
using its slope and y-intercept.
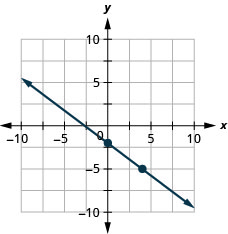
Graph the line of the equation ![]() using its slope and y-intercept.
using its slope and y-intercept.
Graph the line of the equation ![]() using its slope and y-intercept.
using its slope and y-intercept.
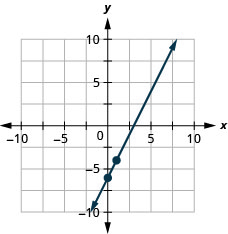
Graph the line of the equation ![]() using its slope and y-intercept.
using its slope and y-intercept.
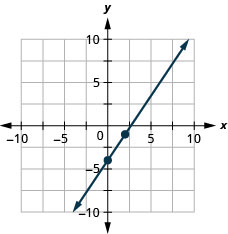
We accept used a filigree with ![]() and
and ![]() both going from nigh
both going from nigh ![]() to 10 for all the equations we've graphed so far. Not all linear equations can exist graphed on this small grid. Often, especially in applications with real-world data, nosotros'll need to extend the axes to bigger positive or smaller negative numbers.
to 10 for all the equations we've graphed so far. Not all linear equations can exist graphed on this small grid. Often, especially in applications with real-world data, nosotros'll need to extend the axes to bigger positive or smaller negative numbers.
Graph the line of the equation ![]() using its gradient and y-intercept.
using its gradient and y-intercept.
Graph the line of the equation ![]() using its slope and y-intercept.
using its slope and y-intercept.
Graph the line of the equation ![]() using its slope and y-intercept.
using its slope and y-intercept.
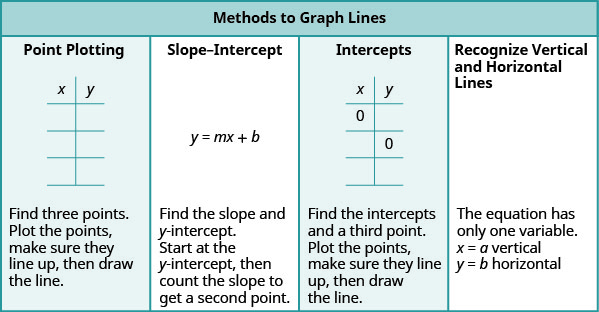
At present that nosotros have graphed lines by using the slope and y-intercept, let's summarize all the methods nosotros have used to graph lines. Come across (Figure).
Choose the Nigh Convenient Method to Graph a Line
Now that we have seen several methods we tin use to graph lines, how practise we know which method to use for a given equation?
While we could plot points, apply the slope–intercept grade, or notice the intercepts for any equation, if we recognize the near convenient way to graph a sure type of equation, our work volition exist easier. Generally, plotting points is not the most efficient way to graph a line. We saw better methods in sections 4.iii, iv.four, and earlier in this section. Let's look for some patterns to help determine the most convenient method to graph a line.
Here are half-dozen equations nosotros graphed in this chapter, and the method we used to graph each of them.

Equations #1 and #two each take but ane variable. Remember, in equations of this grade the value of that ane variable is constant; it does non depend on the value of the other variable. Equations of this grade have graphs that are vertical or horizontal lines.
In equations #iii and #4, both ![]() and
and ![]() are on the aforementioned side of the equation. These two equations are of the course
are on the aforementioned side of the equation. These two equations are of the course ![]() . We substituted
. We substituted ![]() to find the x-intercept and
to find the x-intercept and ![]() to find the y-intercept, and then plant a third point by choosing another value for
to find the y-intercept, and then plant a third point by choosing another value for ![]() or
or ![]() .
.
Equations #5 and #6 are written in slope–intercept course. After identifying the slope and y-intercept from the equation nosotros used them to graph the line.
This leads to the following strategy.
Strategy for Choosing the Nearly Convenient Method to Graph a Line
Consider the form of the equation.
Determine the well-nigh user-friendly method to graph each line.
ⓐ ![]() ⓑ
ⓑ ![]() ⓒ
ⓒ ![]() ⓓ
ⓓ ![]() .
.
Determine the virtually user-friendly method to graph each line: ⓐ ![]() ⓑ
ⓑ ![]() ⓒ
ⓒ ![]() ⓓ
ⓓ ![]() .
.
ⓐ interceptsⓑ horizontal lineⓒ gradient–interceptⓓ vertical line
Determine the nearly convenient method to graph each line: ⓐ ![]() ⓑ
ⓑ ![]() ⓒ
ⓒ ![]() ⓓ
ⓓ ![]() .
.
ⓐ vertical lineⓑ slope–interceptⓒ horizontal lineⓓ intercepts
Graph and Translate Applications of Slope–Intercept
Many real-world applications are modeled by linear equations. We volition take a look at a few applications here and so you can come across how equations written in slope–intercept grade relate to existent-globe situations.
Usually when a linear equation models a real-world situation, different letters are used for the variables, instead of x and y. The variable names remind united states of america of what quantities are existence measured.
The equation ![]() is used to catechumen temperatures,
is used to catechumen temperatures, ![]() , on the Celsius scale to temperatures,
, on the Celsius scale to temperatures, ![]() , on the Fahrenheit scale.
, on the Fahrenheit scale.
ⓐ Find the Fahrenheit temperature for a Celsius temperature of 0.
ⓑ Find the Fahrenheit temperature for a Celsius temperature of 20.
ⓒ Interpret the slope and F-intercept of the equation.
ⓓ Graph the equation.
Solution
| ⓐ Detect the Fahrenheit temperature for a Celsius temperature of 0. Find Simplify. | 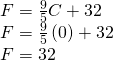 |
| ⓑ Find the Fahrenheit temperature for a Celsius temperature of xx. Find Simplify. Simplify. |  |
ⓒ Interpret the slope and F-intercept of the equation.
Fifty-fifty though this equation uses ![]() and
and ![]() , information technology is still in gradient–intercept form.
, information technology is still in gradient–intercept form.

The slope, ![]() , means that the temperature Fahrenheit (F) increases 9 degrees when the temperature Celsius (C) increases 5 degrees.
, means that the temperature Fahrenheit (F) increases 9 degrees when the temperature Celsius (C) increases 5 degrees.
The F-intercept means that when the temperature is ![]() on the Celsius scale, it is
on the Celsius scale, it is ![]() on the Fahrenheit calibration.
on the Fahrenheit calibration.
ⓓ Graph the equation.
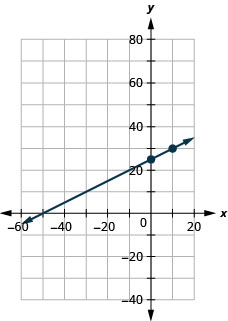
Nosotros'll need to use a larger scale than our usual. Beginning at the F-intercept ![]() then count out the rise of 9 and the run of 5 to get a second point. Run into (Figure).
then count out the rise of 9 and the run of 5 to get a second point. Run into (Figure).
The equation ![]() is used to gauge a woman'south top in inches, h, based on her shoe size, s.
is used to gauge a woman'south top in inches, h, based on her shoe size, s.
ⓐ Estimate the peak of a kid who wears women's shoe size 0.
ⓑ Estimate the top of a woman with shoe size viii.
ⓒ Interpret the slope and h-intercept of the equation.
ⓓ Graph the equation.
- ⓐ 50 inches
- ⓑ 66 inches
- ⓒ The slope, 2, means that the height, h, increases past ii inches when the shoe size, s, increases past one. The h-intercept means that when the shoe size is 0, the acme is 50 inches.
- ⓓ

The equation ![]() is used to judge the temperature in degrees Fahrenheit, T, based on the number of cricket chirps, due north, in one infinitesimal.
is used to judge the temperature in degrees Fahrenheit, T, based on the number of cricket chirps, due north, in one infinitesimal.
ⓐ Gauge the temperature when there are no chirps.
ⓑ Estimate the temperature when the number of chirps in one infinitesimal is 100.
ⓒ Interpret the gradient and T-intercept of the equation.
ⓓ Graph the equation.
The price of running some types business has two components—a fixed cost and a variable cost. The fixed price is always the same regardless of how many units are produced. This is the cost of rent, insurance, equipment, advertizement, and other items that must be paid regularly. The variable cost depends on the number of units produced. It is for the material and labor needed to produce each item.
Stella has a home business concern selling gourmet pizzas. The equation ![]() models the relation between her weekly cost, C, in dollars and the number of pizzas, p, that she sells.
models the relation between her weekly cost, C, in dollars and the number of pizzas, p, that she sells.
ⓐ Find Stella's cost for a week when she sells no pizzas.
ⓑ Find the cost for a calendar week when she sells 15 pizzas.
ⓒ Translate the slope and C-intercept of the equation.
ⓓ Graph the equation.
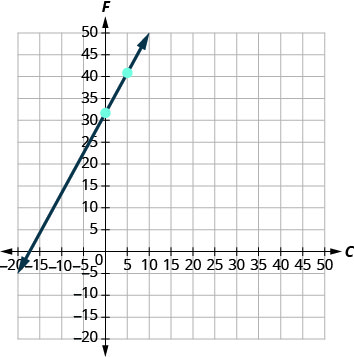
Sam drives a delivery van. The equation ![]() models the relation between his weekly cost, C, in dollars and the number of miles, thou, that he drives.
models the relation between his weekly cost, C, in dollars and the number of miles, thou, that he drives.
ⓐ Notice Sam's price for a week when he drives 0 miles.
ⓑ Notice the cost for a week when he drives 250 miles.
ⓒ Interpret the slope and C-intercept of the equation.
ⓓ Graph the equation.
- ⓐ ?60
- ⓑ ?185
- ⓒ The slope, 0.5, means that the weekly price, C, increases past ?0.50 when the number of miles driven, n, increases by 1. The C-intercept means that when the number of miles driven is 0, the weekly cost is ?60
- ⓓ
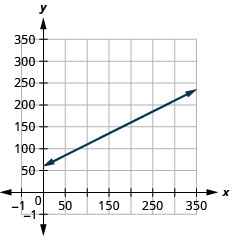
Loreen has a calligraphy business organization. The equation ![]() models the relation between her weekly cost, C, in dollars and the number of wedding ceremony invitations, northward, that she writes.
models the relation between her weekly cost, C, in dollars and the number of wedding ceremony invitations, northward, that she writes.
ⓐ Find Loreen's toll for a week when she writes no invitations.
ⓑ Find the cost for a week when she writes 75 invitations.
ⓒ Translate the slope and C-intercept of the equation.
ⓓ Graph the equation.
- ⓐ ?35
- ⓑ ?170
- ⓒ The slope, i.viii, means that the weekly price, C, increases by ?1.80 when the number of invitations, north, increases past 1.fourscore.
The C-intercept ways that when the number of invitations is 0, the weekly cost is ?35.; - ⓓ
Use Slopes to Place Parallel Lines
The gradient of a line indicates how steep the line is and whether information technology rises or falls as nosotros read it from left to correct. Ii lines that take the same slope are chosen parallel lines. Parallel lines never intersect.
We say this more formally in terms of the rectangular coordinate system. Ii lines that accept the aforementioned slope and different y-intercepts are chosen parallel lines. See (Figure).
Verify that both lines have the same gradient, ![]() , and different y-intercepts.
, and different y-intercepts.
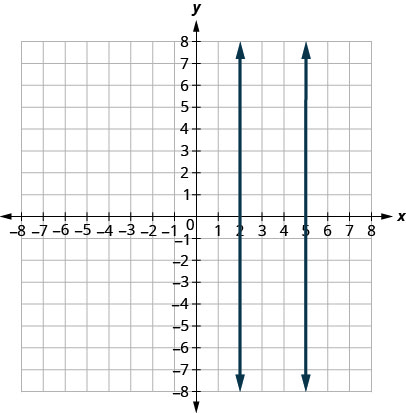
What nigh vertical lines? The slope of a vertical line is undefined, and then vertical lines don't fit in the definition in a higher place. We say that vertical lines that have different x-intercepts are parallel. See (Figure).
Vertical lines with diferent x-intercepts are parallel.
Parallel Lines
Parallel lines are lines in the same airplane that exercise not intersect.
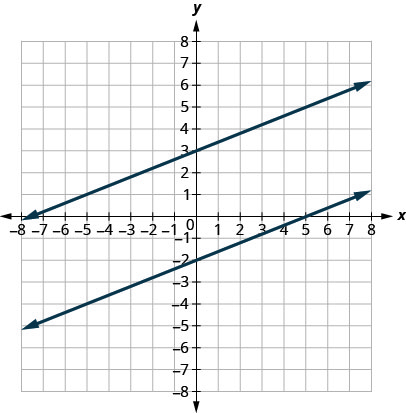
Let's graph the equations ![]() and
and ![]() on the aforementioned filigree. The offset equation is already in slope–intercept grade:
on the aforementioned filigree. The offset equation is already in slope–intercept grade: ![]() . We solve the 2nd equation for
. We solve the 2nd equation for ![]() :
:
![]()
Graph the lines.
Observe the lines look parallel. What is the slope of each line? What is the y-intercept of each line?

The slopes of the lines are the same and the y-intercept of each line is dissimilar. So we know these lines are parallel.
Since parallel lines accept the same slope and different y-intercepts, nosotros can now just look at the slope–intercept course of the equations of lines and decide if the lines are parallel.
Use slopes and y-intercepts to determine if the lines ![]() and
and ![]() are parallel.
are parallel.
Apply slopes and y-intercepts to determine if the lines ![]() are parallel.
are parallel.
parallel
Apply slopes and y-intercepts to determine if the lines ![]() are parallel.
are parallel.
parallel
Use slopes and y-intercepts to determine if the lines ![]() and
and ![]() are parallel.
are parallel.
Utilize slopes and y-intercepts to decide if the lines ![]() are parallel.
are parallel.
parallel
Use slopes and y-intercepts to determine if the lines ![]() are parallel.
are parallel.
parallel
Utilise slopes and y-intercepts to determine if the lines ![]() and
and ![]() are parallel.
are parallel.
Solution
![]()
Since in that location is no![]() , the equations cannot exist put in slope–intercept course. But we recognize them equally equations of vertical lines. Their x-intercepts are
, the equations cannot exist put in slope–intercept course. But we recognize them equally equations of vertical lines. Their x-intercepts are ![]() and
and ![]() . Since their x-intercepts are different, the vertical lines are parallel.
. Since their x-intercepts are different, the vertical lines are parallel.
Use slopes and y-intercepts to make up one's mind if the lines ![]() and
and ![]() are parallel.
are parallel.
parallel
Employ slopes and y-intercepts to determine if the lines ![]() and
and ![]() are parallel.
are parallel.
parallel
Utilise slopes and y-intercepts to decide if the lines ![]() and
and ![]() are parallel. You lot may want to graph these lines, too, to see what they look like.
are parallel. You lot may want to graph these lines, too, to see what they look like.
Employ slopes and y-intercepts to determine if the lines ![]() and
and ![]() are parallel.
are parallel.
not parallel; same line
Use slopes and y-intercepts to decide if the lines ![]() and
and ![]() are parallel.
are parallel.
not parallel; same line
Use Slopes to Identify Perpendicular Lines
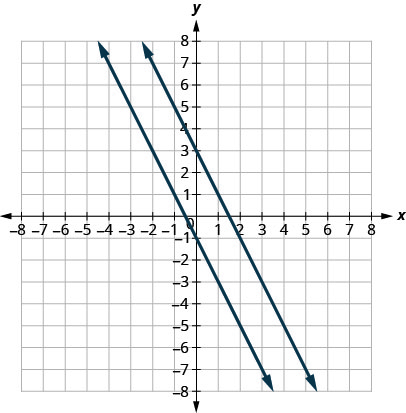
Let's expect at the lines whose equations are ![]() and
and ![]() , shown in (Figure).
, shown in (Figure).

These lines lie in the same airplane and intersect in right angles. We phone call these lines perpendicular.
What practice you notice about the slopes of these two lines? Equally we read from left to right, the line ![]() rises, so its slope is positive. The line
rises, so its slope is positive. The line![]() drops from left to right, so it has a negative slope. Does it brand sense to you that the slopes of two perpendicular lines will have reverse signs?
drops from left to right, so it has a negative slope. Does it brand sense to you that the slopes of two perpendicular lines will have reverse signs?
If we expect at the gradient of the offset line, ![]() , and the slope of the second line,
, and the slope of the second line, ![]() , nosotros can see that they are negative reciprocals of each other. If we multiply them, their production is
, nosotros can see that they are negative reciprocals of each other. If we multiply them, their production is ![]()
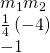
This is always truthful for perpendicular lines and leads us to this definition.
Perpendicular Lines
Perpendicular lines are lines in the same airplane that form a right bending.
If ![]() are the slopes of 2 perpendicular lines, then:
are the slopes of 2 perpendicular lines, then:
![]()
Vertical lines and horizontal lines are always perpendicular to each other.
We were able to look at the slope–intercept form of linear equations and make up one's mind whether or not the lines were parallel. We tin exercise the same matter for perpendicular lines.
We find the slope–intercept form of the equation, and then encounter if the slopes are negative reciprocals. If the production of the slopes is ![]() , the lines are perpendicular. Perpendicular lines may have the same y-intercepts.
, the lines are perpendicular. Perpendicular lines may have the same y-intercepts.
Use slopes to decide if the lines, ![]() and
and ![]() are perpendicular.
are perpendicular.
Solution
| The first equation is already in gradient-intercept form. | | |
| Solve the second equation for |  | |
| Identify the slope of each line. | 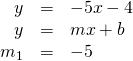 |  |
The slopes are negative reciprocals of each other, so the lines are perpendicular. We check past multiplying the slopes,
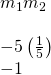
Use slopes to determine if the lines ![]() and
and ![]() are perpendicular.
are perpendicular.
perpendicular
Utilize slopes to determine if the lines ![]() and
and ![]() are perpendicular.
are perpendicular.
perpendicular
Employ slopes to make up one's mind if the lines, ![]() and
and ![]() are perpendicular.
are perpendicular.
Use slopes to determine if the lines ![]() and
and ![]() are perpendicular.
are perpendicular.
non perpendicular
Utilize slopes to determine if the lines ![]() and
and ![]() are perpendicular.
are perpendicular.
not perpendicular
Fundamental Concepts
Practice Makes Perfect
Recognize the Relation Between the Graph and the Slope–Intercept Form of an Equation of a Line
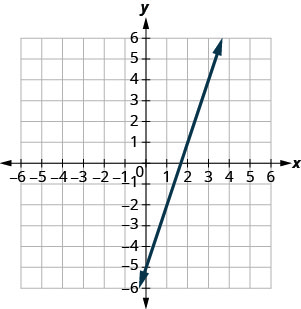
In the following exercises, use the graph to find the slope and y-intercept of each line. Compare the values to the equation ![]() .
.
![]()
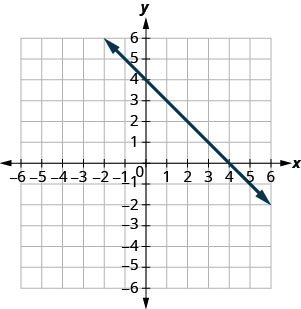
![]()
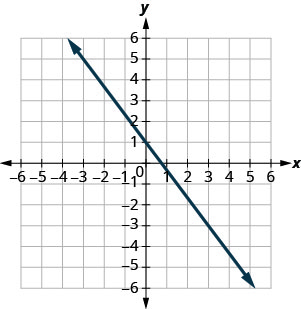
![]()
Identify the Slope and y-Intercept From an Equation of a Line
In the following exercises, place the slope and y-intercept of each line.
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
Graph a Line Using Its Gradient and Intercept
In the following exercises, graph the line of each equation using its slope and y-intercept.
![]()
![]()
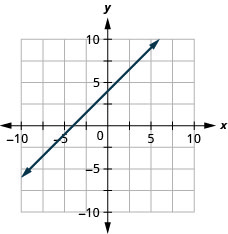
![]()
![]()

![]()
![]()
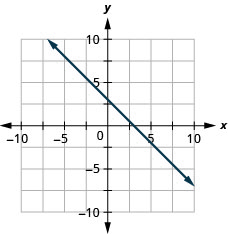
![]()
![]()
![]()
![]()

![]()
![]()
![]()
![]()

![]()
![]()
Choose the Most Convenient Method to Graph a Line
In the following exercises, determine the almost convenient method to graph each line.
![]()
![]()
horizontal line
![]()
![]()
vertical line
![]()
![]()
slope–intercept
![]()
![]()
intercepts
![]()
![]()
slope–intercept
![]()
![]()
horizontal line
![]()
![]()
intercepts
![]()
![]()
gradient–intercept
Graph and Interpret Applications of Slope–Intercept
The equation ![]() models the relation betwixt the amount of Tuyet's monthly water bill payment, P, in dollars, and the number of units of water, westward, used.
models the relation betwixt the amount of Tuyet's monthly water bill payment, P, in dollars, and the number of units of water, westward, used.
- ⓐ Detect Tuyet'south payment for a month when 0 units of h2o are used.
- ⓑ Find Tuyet'south payment for a calendar month when 12 units of water are used.
- ⓒ Translate the slope and P-intercept of the equation.
- ⓓ Graph the equation.
The equation ![]() models the relation between the amount of Randy's monthly water neb payment, P, in dollars, and the number of units of water, w, used.
models the relation between the amount of Randy's monthly water neb payment, P, in dollars, and the number of units of water, w, used.
- ⓐ Find the payment for a month when Randy used 0 units of h2o.
- ⓑ Find the payment for a month when Randy used fifteen units of water.
- ⓒ Translate the gradient and P-intercept of the equation.
- ⓓ Graph the equation.
- ⓐ ?28
- ⓑ ?66.10
- ⓒ The gradient, 2.54, means that Randy's payment, P, increases by ?two.54 when the number of units of water he used, w, increases by one. The P–intercept ways that if the number units of water Randy used was 0, the payment would be ?28.
- ⓓ
Bruce drives his motorcar for his job. The equation ![]() models the relation between the corporeality in dollars, R, that he is reimbursed and the number of miles, thou, he drives in 1 mean solar day.
models the relation between the corporeality in dollars, R, that he is reimbursed and the number of miles, thou, he drives in 1 mean solar day.
- ⓐ Observe the amount Bruce is reimbursed on a 24-hour interval when he drives 0 miles.
- ⓑ Find the amount Bruce is reimbursed on a 24-hour interval when he drives 220 miles.
- ⓒ Interpret the gradient and R-intercept of the equation.
- ⓓ Graph the equation.
Janelle is planning to hire a auto while on vacation. The equation ![]() models the relation betwixt the cost in dollars, C, per day and the number of miles, m, she drives in one day.
models the relation betwixt the cost in dollars, C, per day and the number of miles, m, she drives in one day.
- ⓐ Find the cost if Janelle drives the car 0 miles 1 mean solar day.
- ⓑ Notice the toll on a solar day when Janelle drives the car 400 miles.
- ⓒ Translate the slope and C–intercept of the equation.
- ⓓ Graph the equation.
- ⓐ ?15
- ⓑ ?143
- ⓒ The gradient, 0.32, means that the cost, C, increases past ?0.32 when the number of miles driven, m, increases by i. The C-intercept means that if Janelle drives 0 miles one day, the price would be ?15.
- ⓓ
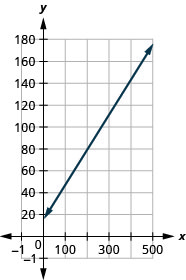
Cherie works in retail and her weekly bacon includes committee for the amount she sells. The equation ![]() models the relation between her weekly salary, S, in dollars and the amount of her sales, c, in dollars.
models the relation between her weekly salary, S, in dollars and the amount of her sales, c, in dollars.
- ⓐ Notice Cherie's salary for a week when her sales were 0.
- ⓑ Find Cherie'southward salary for a week when her sales were 3600.
- ⓒ Interpret the slope and Due south–intercept of the equation.
- ⓓ Graph the equation.
Patel's weekly salary includes a base pay plus commission on his sales. The equation ![]() models the relation between his weekly bacon, S, in dollars and the amount of his sales, c, in dollars.
models the relation between his weekly bacon, S, in dollars and the amount of his sales, c, in dollars.
- ⓐ Find Patel'southward salary for a week when his sales were 0.
- ⓑ Find Patel'south salary for a week when his sales were 18,540.
- ⓒ Translate the gradient and S-intercept of the equation.
- ⓓ Graph the equation.
- ⓐ ?750
- ⓑ ?2418.lx
- ⓒ The slope, 0.09, means that Patel'south salary, S, increases past ?0.09 for every ?i increment in his sales. The Southward-intercept means that when his sales are ?0, his salary is ?750.
- ⓓ
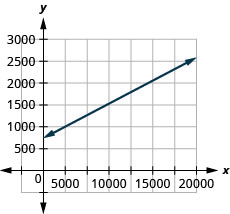
Costa is planning a lunch banquet. The equation ![]() models the relation between the cost in dollars, C, of the feast and the number of guests, g.
models the relation between the cost in dollars, C, of the feast and the number of guests, g.
- ⓐ Observe the cost if the number of guests is 40.
- ⓑ Discover the toll if the number of guests is fourscore.
- ⓒ Interpret the slope and C-intercept of the equation.
- ⓓ Graph the equation.
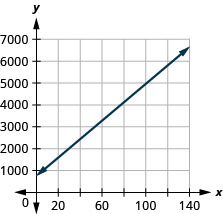
Margie is planning a dinner banquet. The equation ![]() models the relation between the toll in dollars, C of the feast and the number of guests, g.
models the relation between the toll in dollars, C of the feast and the number of guests, g.
- ⓐ Notice the cost if the number of guests is l.
- ⓑ Detect the cost if the number of guests is 100.
- ⓒ Interpret the slope and C–intercept of the equation.
- ⓓ Graph the equation.
- ⓐ ?2850
- ⓑ ?4950
- ⓒ The slope, 42, ways that the toll, C, increases past ?42 for when the number of guests increases past 1. The C-intercept ways that when the number of guests is 0, the cost would be ?750.
- ⓓ
Use Slopes to Identify Parallel Lines
In the post-obit exercises, apply slopes and y-intercepts to decide if the lines are parallel.
![]()
![]()
parallel
![]()
![]()
parallel
![]()
![]()
non parallel
![]()
![]()
parallel
![]()
![]()
parallel
![]()
![]()
parallel
![]()
![]()
parallel
![]()
![]()
parallel
![]()
![]()
not parallel
![]()
![]()
not parallel
![]()
![]()
not parallel
![]()
![]()
non parallel
![]()
![]()
not parallel
Use Slopes to Identify Perpendicular Lines
In the following exercises, use slopes and y-intercepts to determine if the lines are perpendicular.
![]()
![]()
perpendicular
![]()
![]()
perpendicular
![]()
![]()
not perpendicular
![]()
![]()
not perpendicular
![]()
![]()
perpendicular
![]()
![]()
perpendicular
Everyday Math
The equation ![]() can be used to convert temperatures F, on the Fahrenheit scale to temperatures, C, on the Celsius calibration.
can be used to convert temperatures F, on the Fahrenheit scale to temperatures, C, on the Celsius calibration.
- ⓐ Explain what the slope of the equation means.
- ⓑ Explicate what the C–intercept of the equation means.
The equation ![]() is used to guess the number of cricket chirps, n, in one minute based on the temperature in degrees Fahrenheit, T.
is used to guess the number of cricket chirps, n, in one minute based on the temperature in degrees Fahrenheit, T.
- ⓐ Explain what the slope of the equation means.
- ⓑ Explicate what the n–intercept of the equation means. Is this a realistic situation?
Writing Exercises
Explicate in your own words how to decide which method to utilise to graph a line.
Why are all horizontal lines parallel?
Answers will vary.
Self Check
ⓐ Afterwards completing the exercises, apply this checklist to evaluate your mastery of the objectives of this section.

ⓑ After looking at the checklist, do you think you are well-prepared for the adjacent section? Why or why not?
Source: https://opentextbc.ca/elementaryalgebraopenstax/chapter/use-the-slope-intercept-form-of-an-equation-of-a-line/
0 Response to "Review Unit 2a Slope and Slope Intercept Worksheet"
Post a Comment